In concentrated winding, the coil sides of a given phase are concentrated in a single slot under a given pole. the individual coil voltages induced are in phase with each other.
These voltage may be added arithmetically. in order to determine the induce voltage per phase, a given coil voltage is multiplied the number of series connected coils per phase. In actual practice , in each phase coils are not connected in a single slot, but are distributed in a number of slots in space to form a polar group under each pole. the voltage induced in coil sides constituting a polar group are not in phase but differ by an angle equal to the angular displacement β of the slots. the total voltage induce in any phase will be the phasor sum of the individual coil voltage.
In concentrated type all the coil sides will be placed in one slot under a pole. So induced e.m.f. in all the coils will achieve maxima and minima at the same time . all of them will be in phase. Hence resultant e.m.f. after connecting coils in series will be algebraic sum of all the e.m.f.s. as all are in phase.
The distribution factor or breadth factor is defined as the ratio of the actual voltage obtained to possible voltage if all the coils of a polar group were concentrated in a single slot.
Let m = slots per pole per phase , that is slots per phase belt
β = angular displacement between adjacent slots in electrical degrees
Thus, one phase of winding consists of coils arranged in m slots . the voltages Ec1 , Ec2 , Ec3 ....... are the individual coil voltages. each coil voltage E will be out of phase with next coil voltage by the slots pitch β .
Fig show the voltage polygon of the induced voltages in the four coils of a group ( m =4 ) . the voltages Ec1 , Ec2 , Ec3 and Ec4 are represented by phasor AB, BC, CD, and DF respectively in fig. each of these phasor in a chord of a circle centre O and subtends an angle β at O. The phasor sum AF , representing the result winding voltage, subtends an angle mβ at the centre.
Arithmetic sum of individual coil voltages
= mEc = mAB = m(2AB)
= 2mOAsin / AOM = 2mOAsin β/2
Phasor sum of individual coil voltages
= AF = 2AG = 2OAsin / AOG = 2OAsin mβ/2
it is to be noted that the distribution factor Kd for a given number of phases is dependent only on the number of distributed slots under a given pole. It is independent of type of winding , Lap or Wave or number of turns per coil. As the number of slots per pole increases , the distribution factor decreases.

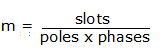



Post a Comment